The Butterfly Effect: A Gateway to Chaos

To grasp chaos theory more effectively, we can rephrase our initial inquiry: What exactly is the Butterfly Effect? This concept is widely recognized and has inspired numerous films. The essence of the Butterfly Effect is that a seemingly minor event, like a butterfly fluttering its wings in Brazil, could trigger a tornado in Texas.

Let’s break this down further. Imagine we’re simulating an electric motor. In this scenario, the butterfly represents an initial condition, perhaps a specific current level. The tornado symbolizes an outcome, which could be an overheated motor. If an electric component draws too much current, overheating is a logical conclusion.

In our simulation, we effectively create an alternate reality by modeling the motor, setting an initial condition, and allowing it to project future outcomes. Now, picture conducting a similar test with a real motor in a vehicle. We supply the same current, but surprisingly, it remains cool. Why? The air circulating around the motor provides adequate cooling, thus contradicting our initial prediction.

This leads us to a fundamental aspect of chaos theory:

Present conditions can dictate future outcomes, yet the approximate present does not accurately determine the future.

Certain systems exhibit a greater degree of chaos, making them particularly challenging to predict. Examples of such chaotic natural systems include:

• Fluid dynamics
• Irregular human heartbeat patterns
• Weather phenomena
• Climate variations

If you’re interested in exploring more about these topics, consider joining our community. Your support helps writers like me continue to share knowledge.

Why Predictions Can Go Awry

To understand why predictions are often elusive, consider another straightforward experiment: a ball thrown in a high arc. We have the equation that outlines its trajectory, along with its initial velocity and launch angle. Shouldn’t we be able to determine where the ball will land? Not necessarily. If a strong headwind interrupts its flight, the ball may land much closer to its starting point than anticipated.

How, then, can we accurately predict future events? Theoretically, we would need a dynamic model that adjusts with every change. In the ball-throwing scenario, we would require a new equation for every moment that accounts for the headwind. This necessity illustrates a significant distinction: a model can only maintain one state, whereas reality comprises multiple states that evolve over time.

I often find visualizing concepts helpful. Regarding chaos theory, I envision a point moving along a time axis.

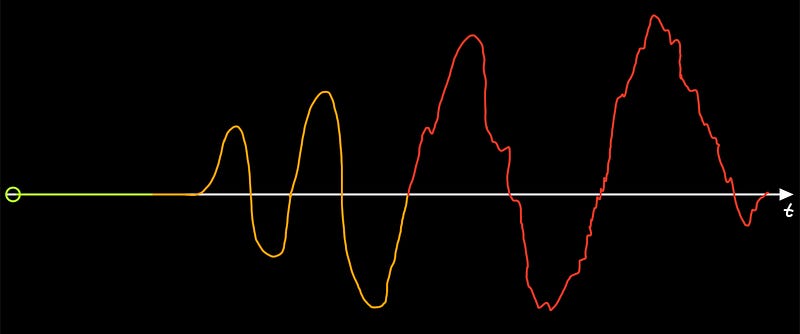
Initially, this point traces a precise line (green), indicating that our model aligns closely with reality. However, as time progresses— or as we extend our predictions— the point increasingly diverges from this line (orange). While our forecasts remain somewhat useful, they become progressively less accurate. Eventually, the divergence becomes so significant that the results lose their relevance, necessitating the cessation of the simulation.

In another light, we can assert that the longer our model runs, the more it strays from the actual situation. This is evident in natural systems, but what about human-made ones? Are there no predictable processes? Unfortunately, there are chaotic systems in our artificial world as well, such as:

• Stock markets
• Road traffic patterns

The chaotic nature of the stock market introduces inherent risks in investing. While thorough research can help minimize these risks, they can never be entirely eliminated. Additionally, I would argue that human society itself embodies chaos. Though characters like Hari Seldon in fiction may predict societal trends, creating a comprehensive model for an entire society—let alone an individual’s future—remains a daunting challenge.

How could anyone have foreseen 27 years ago that a person would pursue a medical career due to a pandemic?